Verify that x^2 cy^2 = 1 is an implicit solution to \frac {dy} {dx} = \frac {xy} {x^2 1} If you're assuming the solution is defined and differentiable for x=0, then one necessarily has y (0)=0 In this case, one can easily identify two trivial solutions, y=x and y=x If you're assuming the solution is defined andApplying Green's Theorem where D is given by the interior of C, ie D is the ellipse such that x2/4y2 ≤ 1 Z C (3x−5y)dx (x 6y)dy = Z Z D (1 −(−5))dxdy = 6 Z Z D 1dxdy = 6×(Area of the ellipse) = 6×2π See chapter 2 for calculating the area of an ellipse by change of variables for a double integral (i) Directly Parameterise C by x(t) = 2cost, y(t) = sint, where 0 ≤ t ≤Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more

Ex 9 5 15 Class 12 Find Solution 2xy Y 2 2x 2 Dy Dx 0 When
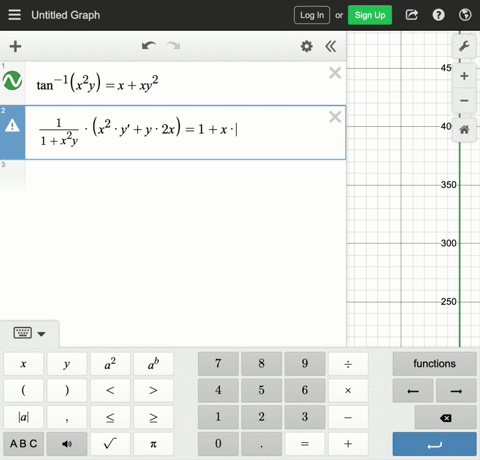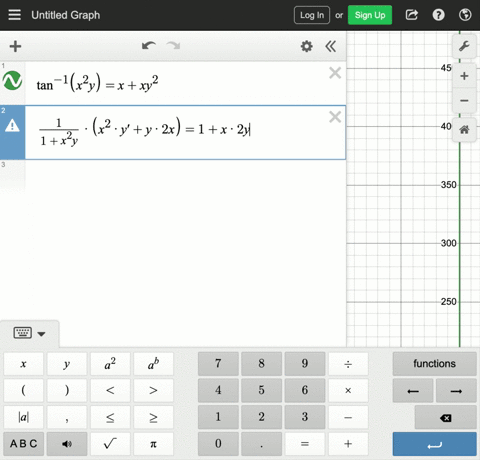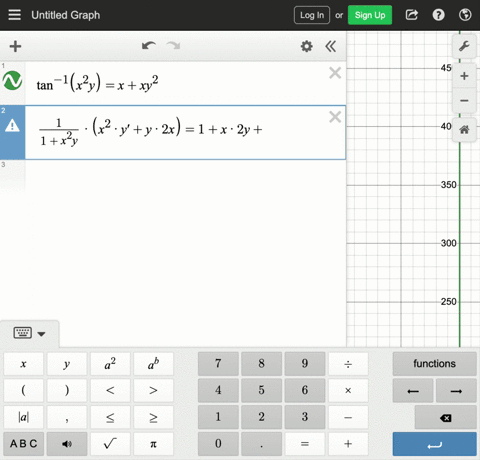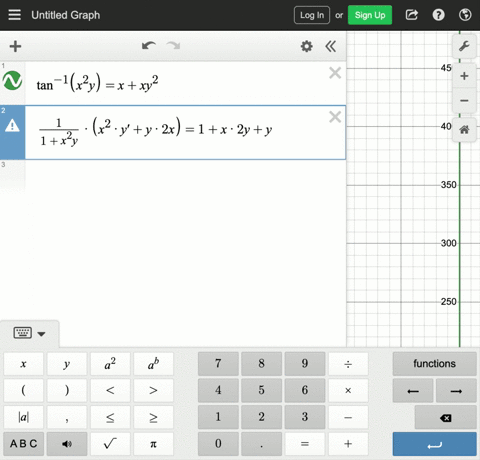
2^x 2^y=2^x y find dy/dx at x=y=1
2^x 2^y=2^x y find dy/dx at x=y=1- Ex 94, 12 Find a particular solution satisfying the given condition 𝑥 𝑥2−1 𝑑𝑦𝑑𝑥=1;𝑦=0 When 𝑥=2 𝑥 𝑥2−1 dy = dx dy = 𝑑𝑥𝑥(𝑥2 − 1) Integrating both sides 𝑑𝑦 = 𝑑𝑥𝑥(𝑥2 − 1) 𝑦 = 𝑑𝑥𝑥(𝑥 1)(𝑥 − 1) We can write integrand as 1𝑥(𝑥 1To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW Solve `(dy)/(dx)=(x^2x y)/(x^2y^2)`



4 2 Implicit Differentiation
Separabledifferentialequationcalculator x\frac{dy}{dx}=y^{2} en Related Symbolab blog posts Advanced Math Solutions – Ordinary Differential Equations Calculator, Separable ODE Last post, we talked about linear first order differential equations In this post, we will talk about separable Let y = y(x) be the solution curve of the differential equation, (y^2 x)dy/dx = 1, satisfying y(0) = 1 asked in Mathematics by Pankaj01 ( 503k points) jee mainSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more
Simple and best practice solution for x^2(y1)dxy^2(x1)dy=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it If y = (x 2)^2/ x, find dy/dx sujatakumaridolly is waiting for your help Add your answer and earn pointsAnswer Rearranging, (dx/dy) = (x/y)^2 x^2 xy Put x=t*y, (dx/dy) = (dt/dy)*y t Putting this back in differential equation, (dt/dy)*y = t^2 (t*y)^2 t*(y^2
Find dy/dx y^2=1/(1x^2) Differentiate both sides of the equation Differentiate the left side of the equation Tap for more steps Differentiate using the chain rule, which states that is where and Tap for more steps To apply the Chain Rule, set as Differentiate using the Power Rule which states that is where Replace all occurrences of with Rewrite as Differentiate the rightCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyDx2 b(x) dy dx c(x)y = f(x) () Note that this method works for nonconstant coefficients a(x), b(x) and c(x) We suppose that we can find two linearly independent solutions to the homogeneous problem Ly = 0, denoted by y 1(x) and y 2(x) We then seek a solution of the inhomogeneous problem () of the form y p(x) = v 1(x)y 1(x)v 2(x)y 2(x) From this assumed form of solution,



Solved Use Implicit Differentiation To Find Dy Dx In Chegg Com



2
To ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW `x(1y^2)dxy(1x^2) dy=0`I think your question is (xy—1)/(xy—2)dy/dx=(xy1)/(xy2)(I) Solutionput (xy)=v then differentiate both sides with respect to 'x' we get 1dy/dx=dv/dxAnswer (1 of 8) \dfrac{dy}{dx}=\dfrac{xy}{xy}\tag*{} \text{Put,} \quad y=vx \implies \dfrac{dy}{dx}= v x\dfrac{dv}{dx} \implies v x\dfrac{dv}{dx}= \dfrac{1v}{1



4 2 Implicit Differentiation



If X 2 Y 2 2 Xy Find Dy Dx Mention Each And Every Step Cbse Class 12 Learn Cbse Forum
If y = cos^1((3x 4√(1 x^2))) find dy/dx asked in Continuity and Differentiability by KumkumBharti (539k points) continuity;0 votes 1 answer Solve the following differential equations √(1 x^2 y^2 x^2y^2) xy(dy/dx) = 0 asked May 11 in Differential Equations by Rachi (297k points)Find dy/dx of y=x^2 2x1 We differentiate each term individually with respect to x the way we differentiate powers of x is x^n goes to nx^ (n1) So x^2 would become 2x, 2x would be just 2 as x^0 is 1 and a constant would just disappear Therefore, dy/dx=2x2 Answered by Vivek M • Maths tutor 670 Views See similar Maths GCSE tutors



2



1
Find dy/dx y = natural log of x^2 y = ln (x2) y = ln ( x 2) Differentiate both sides of the equation d dx (y) = d dx (ln(x2)) d d x ( y) = d d x ( ln ( x 2)) The derivative of y y with respect to x x is y' y ′ y' y ′ Differentiate the right side of the equation Tap for more steps Differentiate using the chain rule, which states thatSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreDx dt = 2x 1− x 2 −xy, dy dt = 3y 1− y 3 −2xy To find the xnullcline, we solve2x 1− x 2 − xy = 0, where multiplying out and collecting the common factor of x gives x(2− x− y) = 0 This gives two xnullclines, the linexy = 2 and the yaxis By plugging in the points (1,0) and (2,2) into2x 1− x 2 − xy, we see that solutions (to the left of the yaxis) move to the right if




Find Dydx Where X 2 Y 2 3xy 1



Implicit Differentiation
Simple and best practice solution for (xyx)dx=(x^2y^2x^2y^21)dy equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve itCalculus Find dy/dx y=1/ (x^2) y = 1 x2 y = 1 x 2 Differentiate both sides of the equation d dx (y) = d dx ( 1 x2) d d x ( y) = d d x ( 1 x 2) The derivative of y y with respect to x x is y' y ′ y' y ′ Differentiate the right side of the equation Tap for more stepsClick here👆to get an answer to your question ️ The solution of dy/dx = x^2 y^2 1/2xy satisfying y(1) = 1 is given by



How To Solve Show The Differential Equations Are Exact 2xy Y Tany Dx X 2 X Tany 2 Secy 2 2 Dy 0 Te Tx 2x Dx Dt Xe Xt 0 Quora




Get Answer Find The Derivative Y 14 5x 2 10x 14 5x 14 10x 10 Find Transtutors
Answer (1 of 2) Given, (x1) dy/dx =x^2 3x 2 dy = { ( x^2 3x 2)/(x1) } dx dy = { (x^2 x 2x 2)/( x 1 )} dx dy = { x(x1)2(x1)/(x1)} dx dy = { (x1Simple and best practice solution for (x^2xyy^2)dx(xy)dy=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve itQuestion find the relation between x and y




Solved Use Implicit Differentiation To Find Dy Dx And Then Chegg Com



If X 2 Y 2 2 Xy Find Dy Dx Sarthaks Econnect Largest Online Education Community
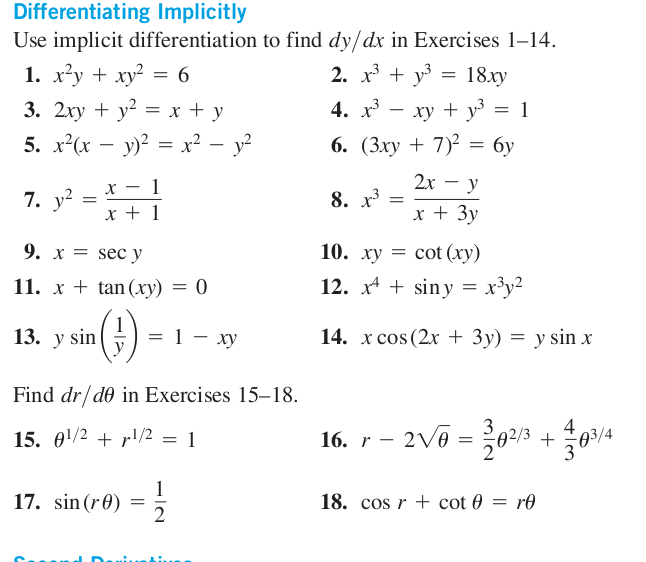
0 votes 1 answer If x = a(cosθ logtanθ/2), y = asinθ , find dy/dx at θ = π/4 asked in Continuity and Differentiability by KumkumBharti (539k points) continuity; If (x2 y2)2 = xy, find dy/dx Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to their queriesHence, du/dx = dy/dx * (2y) This is the derivative of the second component4) For the third component, let w = 2xy We use the product rule dw/dx = 2x*(dy/dx * 1) 2*(y) = dy/dx (2x) 2y This is the derivative of the third component5) Overall the equation differentiates to 2 x ln(2) dy/dx(2y) = dy/dx*(2x) 2y This can be rearranged to



Solving The Bernoulli Differential Equation X 2 Dy Dx Y 2 Xy Youtube



E X Y X Y Find Dy Dx By Implicit Differentiation
Now how did I know that, becuase `dy/dx` is 3x 2 2, y must be the integral of 3x 2 2 `dy/dx` differentiation and integration are opposite processes Ok, so I just integrate this and I get `x^3/3`, and there is 3 on the front, so the 3 cancels out so I just get x 3 2x K This "plus K" is very important Please don't forget it, because it's very important for this sort of problenSimple and best practice solution for dy/dx(2/x2)y=1/x equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it Equation SOLVE Solution for dy/dx(2/x2)y=1/x equation x^521(xy2x)dx(yx2y)dy=0 One solution was found d = 0 Step by step solution Step 1 Step 2 Pulling out like terms 21 Pull out like factors y Is the solution of the math problem right?



Separable Differential Equation Dy Dx Xy 3x Y 3 Xy 2x 4y 8 Youtube




Pure Mathematics Find Dy Dx In Terms Of X And Y When X Y 2xy 3y 2x 7 S O L U T I O N 1 This Is An Implicit Function Because We
Find dy/dx x^2=(xy)/(xy) Differentiate both sides of the equation Differentiate using the Power Rule which states that is where Differentiate the right side of the equation Tap for more steps Differentiate using the Quotient Rule which states that is where and Differentiate Tap for more steps By the Sum Rule, the derivative of with respect to is Differentiate using the PowerLHS log(y) => (1/y)(dy/dx) partial differentiation hence we multiply (1/y) by dy/dx RHS x log(2) => log(2) log(2) is a constant so x dissapears So we get (1/y)(dy/dx) = log(2) 4) We want to find dy/dx, which is on the LHS To get this dy/dx on its own we can multiply both sides by y So we get dy/dx = y log(2) 5) To finish this question we need to sub in for y and then we have an If y = tan1 a/x log (xa/xa) 1/2, prove that dy/dx = 2a 3 /(x 4 – a 4) Mention each and every step Queries asked on Sunday & after 7pm from Monday to Saturday will be answered after 12pm the next working day
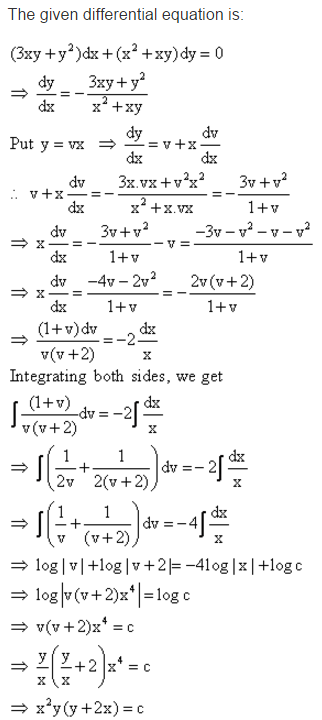



Find The Particular Solution Of Given Differential Equation 3xy Y 2 Dx X 2 Xy Dy 0 At X 1 Y 1 Mathematics Topperlearning Com D1ksg633



Solved Find Dy Dx By Implicit Differentiation 2x 2 Xy Chegg Com
Answer (1 of 4) This is almost certainly a problem in a section of the text on "exact" equations, ie ones of the form dF= F,x dx F,y dy =0 The solution is F(x,y)=constant You need to look for an integrating factor A such that F,x = A 2 x y F,y = A (x^2 y^2 1) Typically if you can'tFind dy/dx y^2(y^24)=x^2(x^25) Differentiate both sides of the equation Differentiate the left side of the equation Tap for more steps Differentiate using the Product Rule which states that is where and By the Sum Rule, the derivative of with respect to is Differentiate using the chain rule, which states that is where and Tap for more steps To apply the Chain Rule, set as dy/dx=x/y x^2y^2=1 Differentiate wrt x d/dxx^2d/dxy^2=d/dx1 We already know how to deal with the first and third terms, so lets get them out the way d/dxx^2d/dxy^2=d/dx1 2xd/dxy^2=0 For the remaining term we use the chain rule, we don't know how to differentiate y^2 wrt x but we do know how to differentiate y^2 wrt y (it the same as differentiating x^2 wrt x!)



Solve The Differential Equation Dy Dx 1 X Y2 Xy2 When Y 0 X 0 Studyrankersonline



How To Find Dy Dx Of This Problem X Siny Xy Quora
BSc MathematicsDifferential EquationDifferential Equation of second order with variable coefficientDifferential Equation(BSc notes) https//wwwyoutube Show that the differential equation dy/dx = y 2 /(xy x 2) is homogeneous and also solve it differential equations;A curve is defined by the parametric equations x = 3^(t) 1, y = 2 x 2^(t) Show that dy\dx = 2 x 3^(2t) Answered by Maleeha K What is the chain rule, product rule and quotient rule and when do I use them?



Solved The General Solution Of Dy Dx Xy 2 X Y Is In Chegg Com




Engineering Mathematics Notes
Misc 14 Find a particular solution of the differential equation (𝑥1) 𝑑𝑦/𝑑𝑥=2 𝑒^(−𝑦)−1 , given that 𝑦=0 when 𝑥=0 (𝑥1) 𝑑𝑦/𝑑𝑥=2𝑒^(−𝑦)−1 The variables are separable 𝑑𝑦/(2𝑒^( −𝑦 ) − 1) = 𝑑𝑥/(𝑥 1) Integrating both sides ∫1 𝑑𝑦/(2𝑒^(−𝑦) − 1) = ∫1 𝑑𝑥/(𝑥 1) ∫1 𝑑𝑦/(2/𝑒^𝑦 − 1Simple and best practice solution for sqrt((1y^2))dxsqrt((1x^2))dy=0 equation Check how easy it is, and learn it for the future Our solution is simple, and easy to understand, so don`t hesitate to use it as a solution of your homework If it's not what You are looking for type in the equation solver your own equation and let us solve it Equation SOLVE Solution for sqrt((1y^2))dxsqrt The general solution of the differential equation (y^2 – x^3 )dx – xydy = 0 (x ≠ 0) is (where c is a constant of integration) asked in Mathematics by Jagan (211k points) jee mains 19;



1




Find A Derivative With Step By Step Math Problem Solver
The nice thing about this differential equation is that the dy dx is already isolated, therefore the answer can be obtained by simply integrating both sides ∫dy = ∫3x 2 x2 dx y = 3 2x2 −2x−1 C y = 3 2x2 − 2 x C 2 = 3 2 (1)2 − 2 1 C 4 − 3 2 = C 5 2 = C Given dy dx = x 1 x2 Multiply both sides by dx dy = x 1 x2 dx Integrate ∫dy = ∫ x 1 x2 dx ∫dy = 1 2 ∫ 2x 1 x2 dx ∫dy = 1 2 du u y = 1 2ln(u) C y = 1 2ln(x2 1) C y = xtan (ln x C) This is a first order linear homogeneous equation NB here homogeneous has its own meaning it means that the equation can be written in form y' = f(x,y) and that f(kx, ky) = f(x,y) for constant k so standard approach is to let v = y/x so y = v * x y' = v' x v thus, plugging this into the original v' x v = v^2 v 1 v' x = v^2 1 (v')/(v^2 1) = 1/(v^2 1




Answered Exexcises E Set 2 Implicit Differentia Bartleby




Worked Example Implicit Differentiation Video Khan Academy
Answered by Hakeem K Solve dy/dx= (x√(x^23))/e^2y given that y=0 when x=1, giving your answer in the form y = f(x) Answered by Ross G We're here to help 44 (0) 3 773Share It On Facebook Twitter Email 1 Answer 1 vote answered by sonuk (445k points) selected by Vikash Kumar Best answer Given differential equation is Thus, the given differential equation is a homogeneous 2power x 2power y =2 power xy find dy /dx Srikarsai1336 Srikarsai1336 Math Secondary School answered 2power x 2power y =2 power xy find dy /dx 2 See answers Advertisement Advertisement




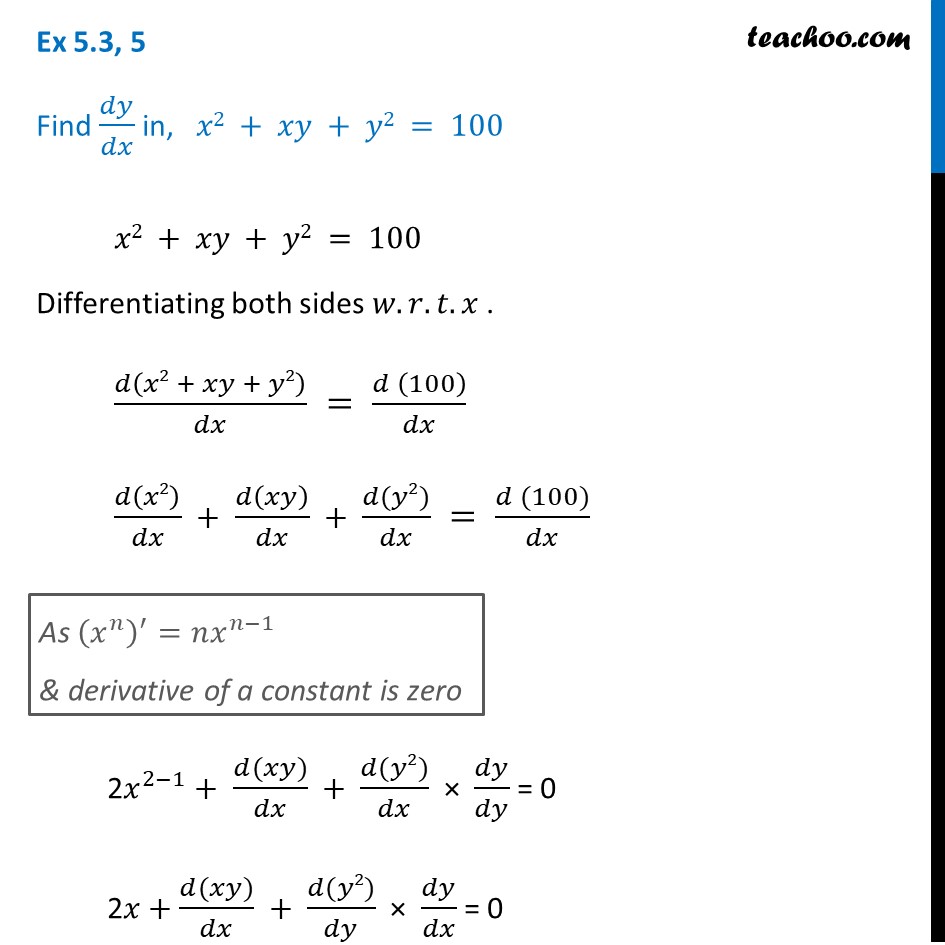
Ex 5 3 5 Find Dy Dx In X2 Xy Y2 100 Class 12



Solved Find Dy Dx By Implicit Differentiation Xy Sqrt X 2 Y 2
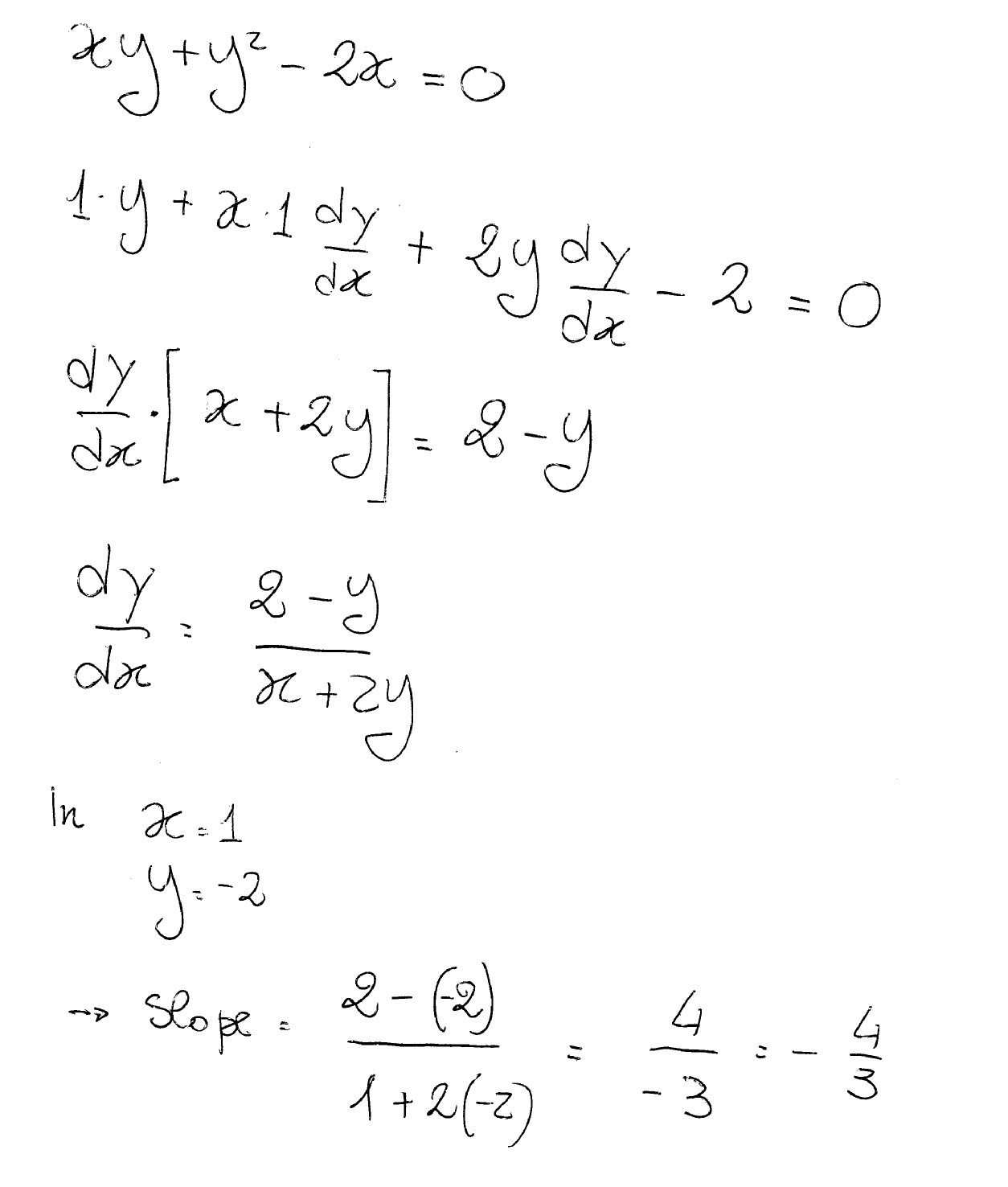



How Do You Differentiate Implicitly Xy Y 2x 0 To Find Dy Dx And Find The Slope Of The Curve At The Given Point 1 2 Socratic



1
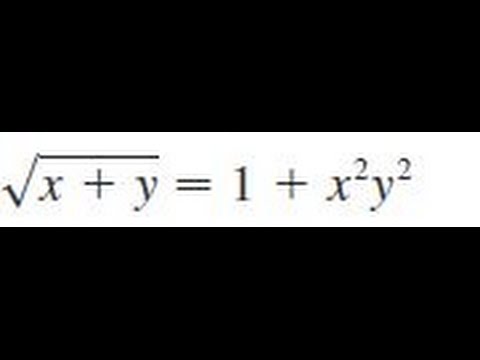



Sqrt X Y 1 X 2y 2 Find Dy Dx By Implicit Differentiation Youtube



3 8 Implicit Differentiation Calculus Volume 1




Solve Dy Dx 2x Y 1 X 2y 3 Youtube
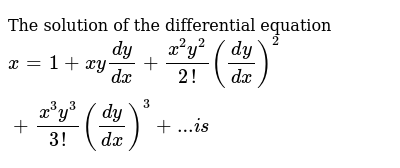



If Dy Dx 7x 2 Y 3 And Y 3 2 Find An Equation For Y In Terms O



How To Homogeneous Reducible Differential Equation Dy Dx 2x Y 1 X 2y 1 Quora
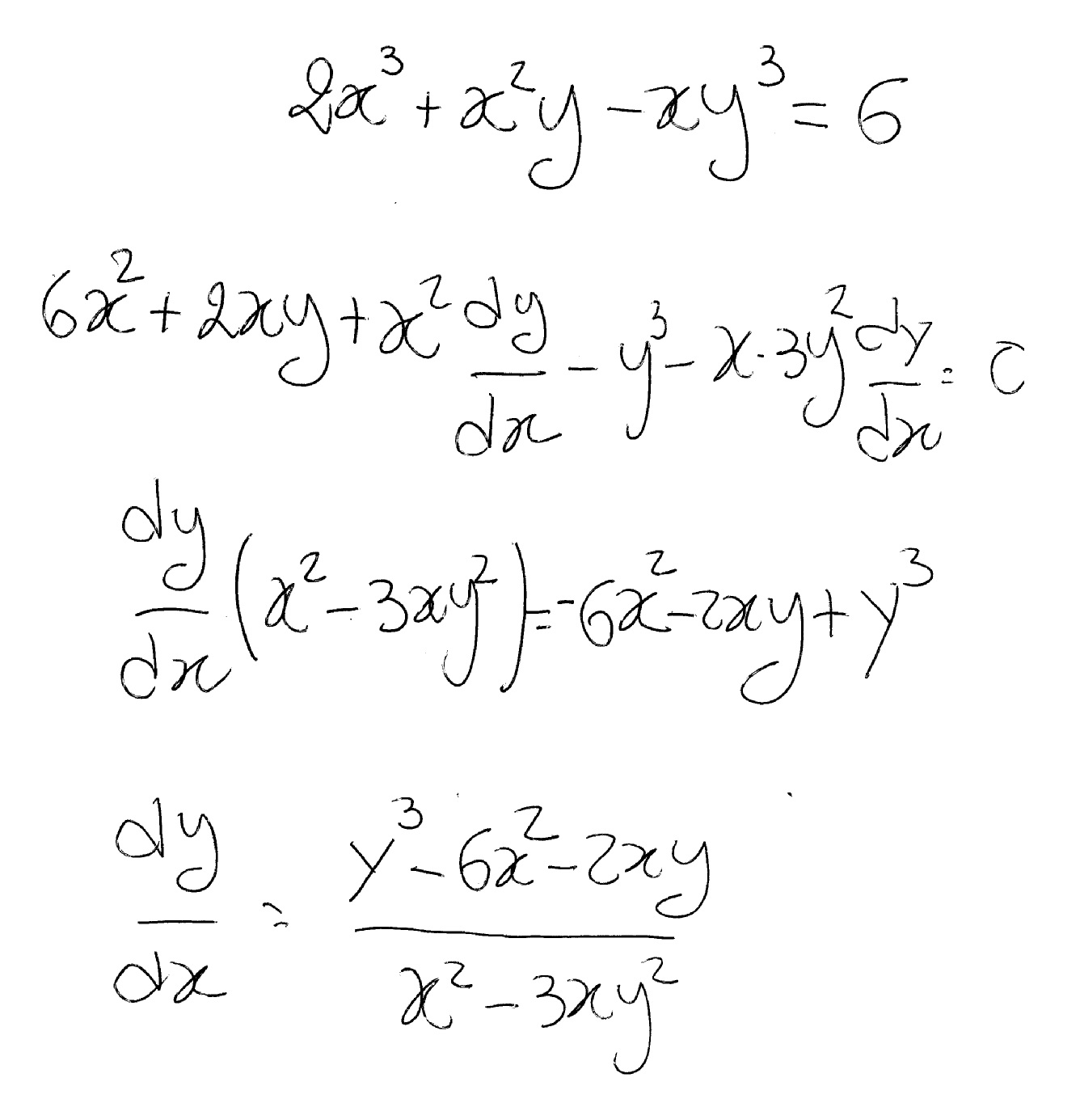



How Do You Find Dy Dx By Implicit Differentiation For 2x 3 X 2 Y Xy 3 6 Socratic



2



Find Dy Dx When X And Y Are Connected By The Relation Sin Xy X Y X2 Y Studyrankersonline




Solved Use Implicit Differentiation To Find Dy Dx And Chegg Com




If 2 X 2 Y 2 X Y Then Dydx Is Equal To




X 2 Y 2 1 Find Dy Dx By Implicit Differentiation Youtube



Ex 5 3 5 Find Dy Dx In X2 Xy Y2 100 Class 12



Find Dy Dx At X 1 Y Pi 4 If Sin 2 Y Cos Xy K Sarthaks Econnect Largest Online Education Community




If E X E Y E X Y Find Dydx




X 2 Y 2 Dx Xydy 0 Happylove22




Ex 9 5 15 Class 12 Find Solution 2xy Y 2 2x 2 Dy Dx 0 When



4 2 Implicit Differentiation



Solved Use Implicit Differentiation To Find Dy Dx Xy X Chegg Com




Solved Differentiating Implicitly Use Implicit Chegg Com




If Y X Is The Solution Of Dy Dx X 2 1 Y 2 Y 0 2 Then Y 3 Homeworklib




12 Math 1 Comm Pages 101 150 Flip Pdf Download Fliphtml5
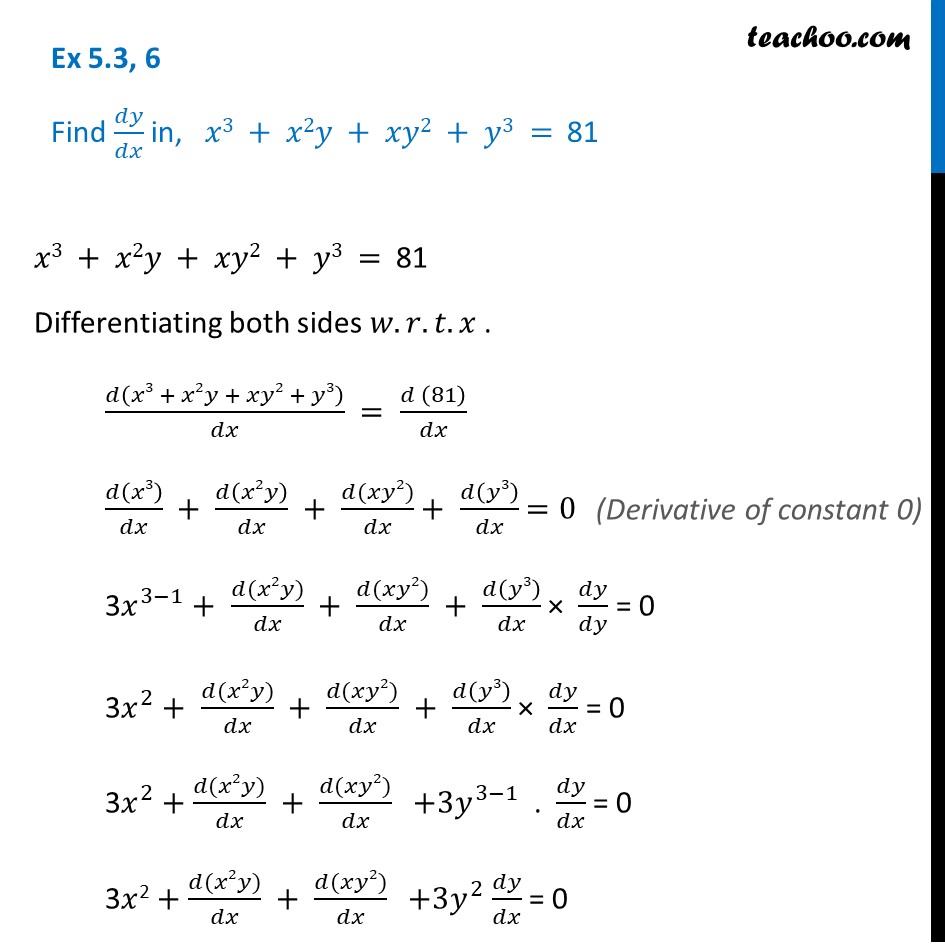



Ex 5 3 6 Find Dy Dx In X3 X2y Xy2 Y3 81 Cbse



2



Points Find Dy Dx For 2 Xy X




Solve Y 2 2x 2y Dx 2x 3 Xy Dy 0 Mathematics Stack Exchange




Find Dy Dx Of X Cos Y Sin X Y Socratic



Solved Find The General Solution To Dy Dx X 2 Y 2 Xy Lebl 1 Course Hero
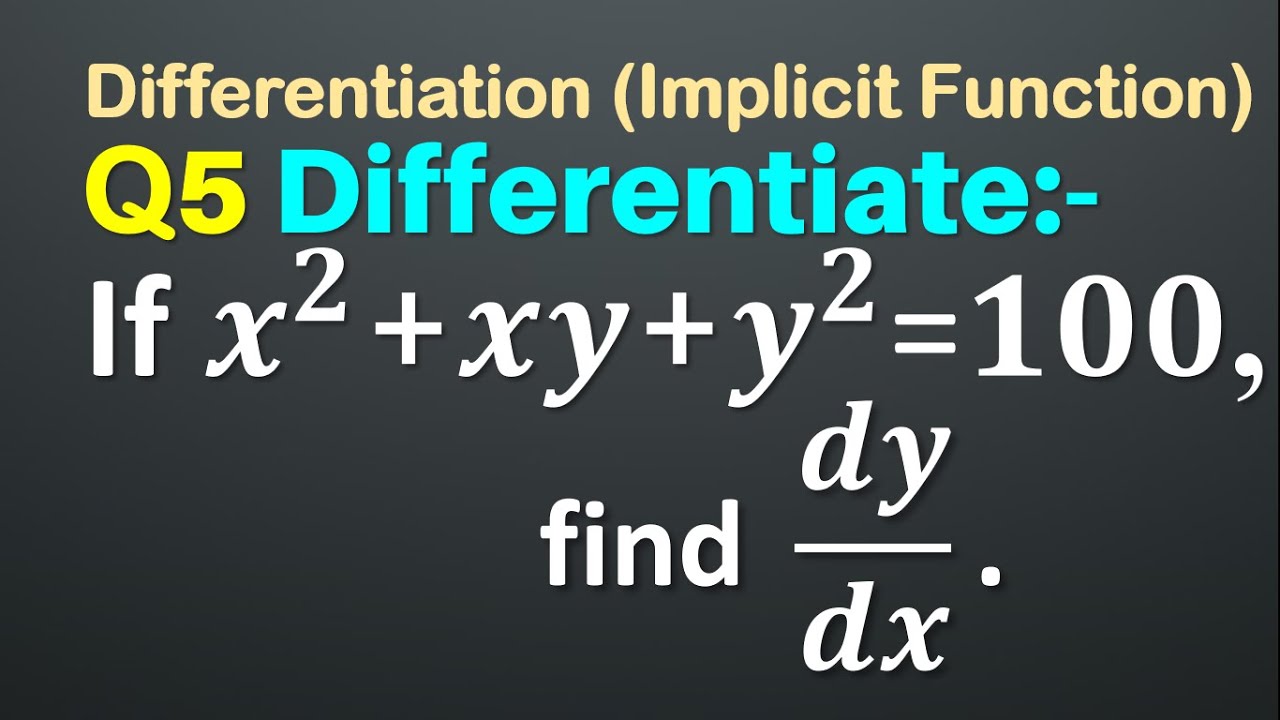



Q5 If X 2 Xy Y 2 100 Find Dy Dx Implicit Function If X2 Xy Y2 100 Find Dy Dx Youtube




Differential Equations Separation Of Variables Ppt Download




Solved Find Dy Dx By Implicit Differentiation X 2 Xy Chegg Com



2
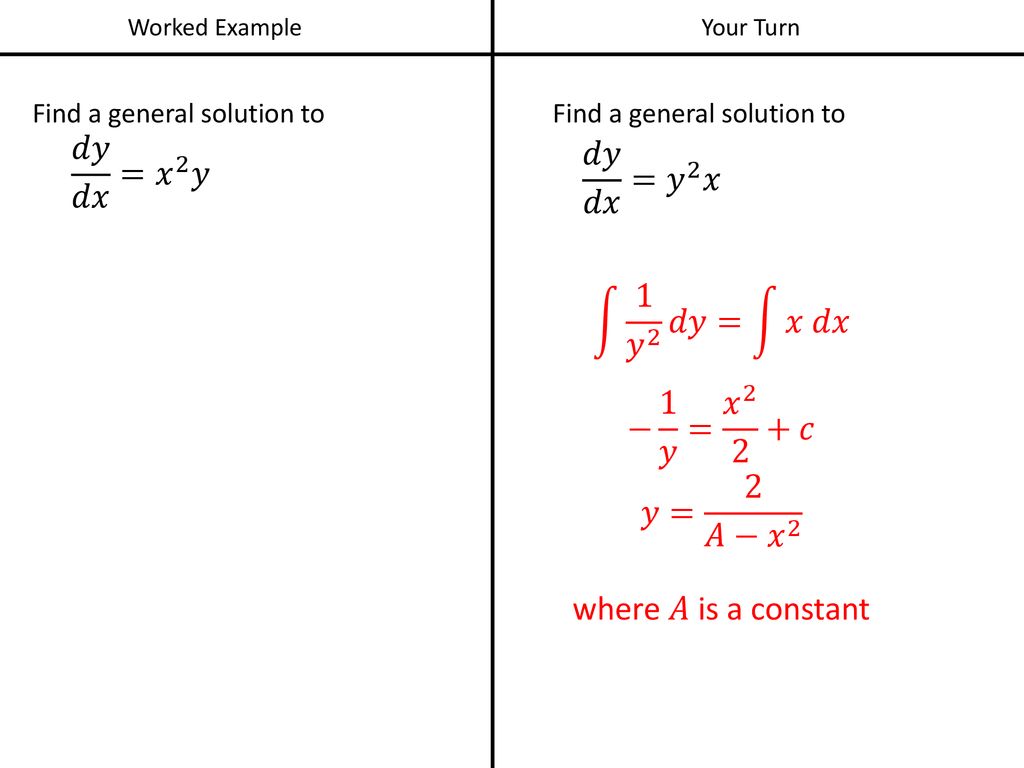



Differential Equations Separation Of Variables Ppt Download



2
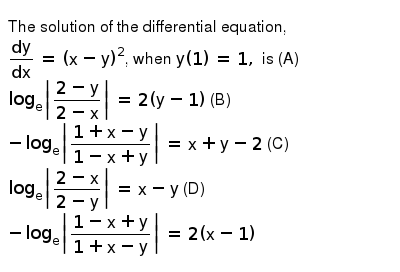



The Solution Of The Differential Equation Dy Dx X Y 2 When Y 1 1 Is A Log E Abs 2 Y 2 X 2 Y 1 B Log E Abs 1 X Y 1 X Y X Y 2 C Log E Abs 2 X 2 Y X Y D Log E Abs 1 X Y 1 X Y 2 X 1




If X2 Y2 2 Xy Find Dy Dx Mention Each And Every Step Mathematics Topperlearning Com Gwvwfskk



Solved Find The General Solution Of The Differential Equation 3 Y X 2 2 X Y Y 3 D X X 2 Y 2 D Y 0 Course Hero




Solve Y 1 Xy Dx X 1 Xy X 2y 2 Dy 0 Mathematics Stack Exchange




If Xy Log X Y 1 Prove That Dydx Y X 2y X Y X Xy 2 X Y




Implicit Differentiation
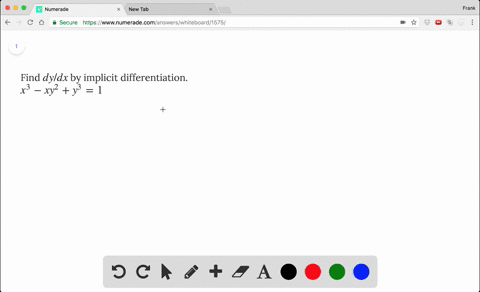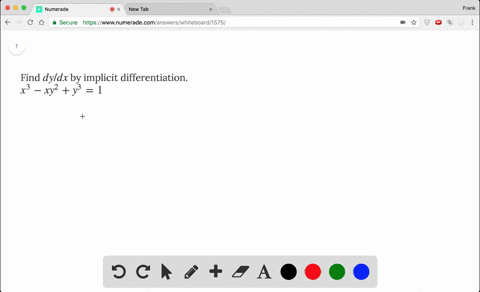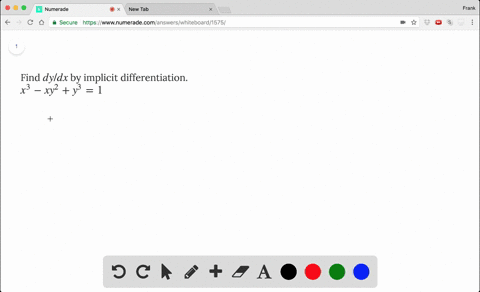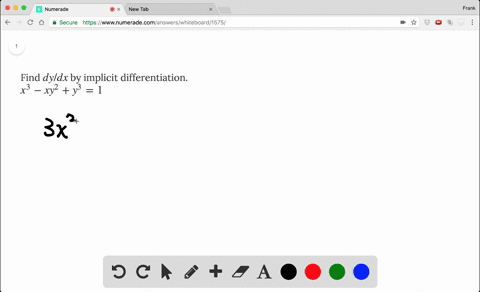



Solved Find Dy Dx By Implicit Differentiation X 3 Xy 2 Y 3 1
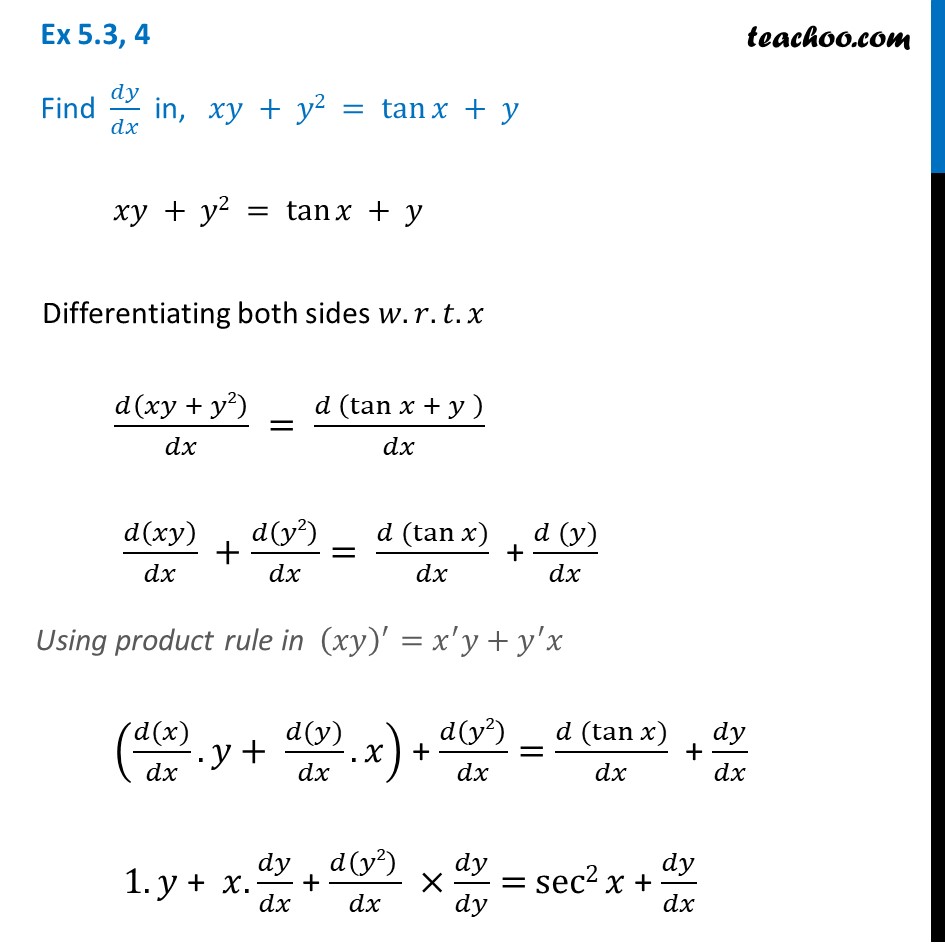



Ex 5 3 4 Find Dy Dx In Xy Y2 Tan X Y Chapter 5



Solved Solve The Following Differential Equations X Y 3 Dx X Y 1 Dy 0 2 X Y 1 Dx 3x 4y 2 Dy C 3 1 Y 2 Xy 2 Dx X 2y Y 2xy Dy Course Hero
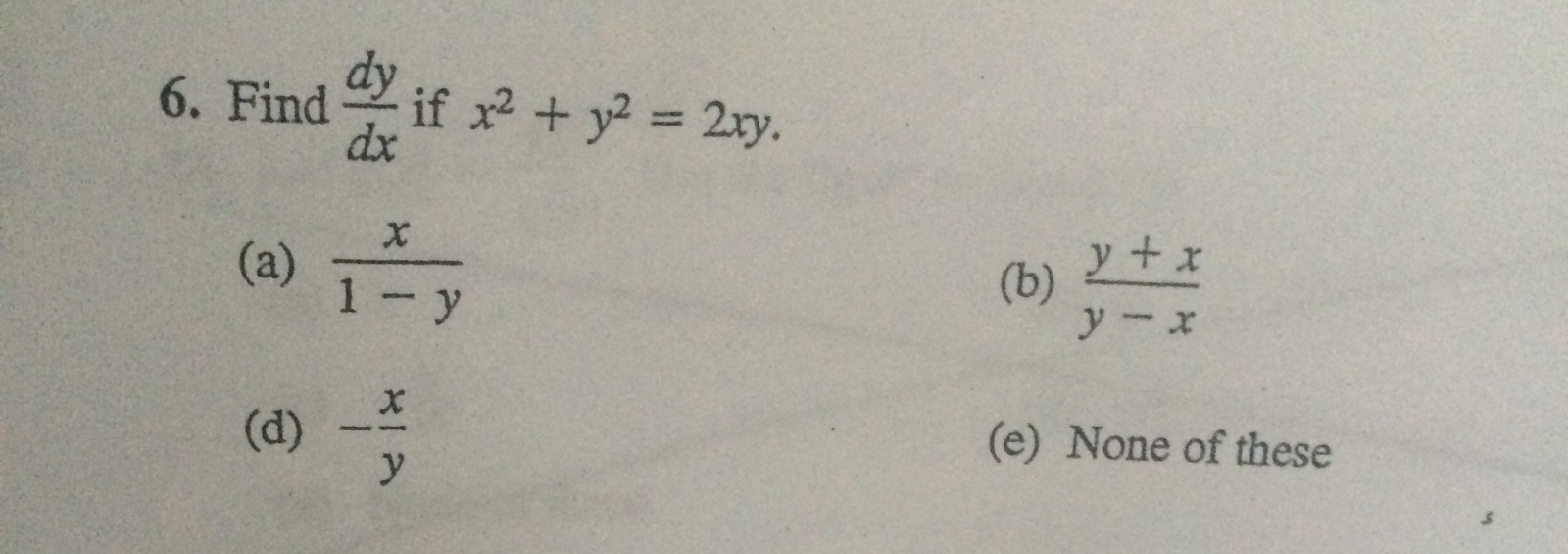



Solved 6 Find Dy Dx If X 2 Y 2 2xy A X 1 Y B Chegg Com
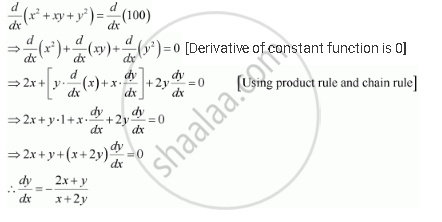



Find Dx Dy For X2 Xy Y2 100 Mathematics Shaalaa Com



Solve Dy Dx Xy 1 X 2 X Y Sarthaks Econnect Largest Online Education Community




Oneclass 1 Use Implicit Differentiation To Find Dy Dx If Xy Sin X Sin Y 2 Suppose 6x2 3xy




Implicit Differentiation Advanced Example Video Khan Academy




Engineering Mathematics Notes
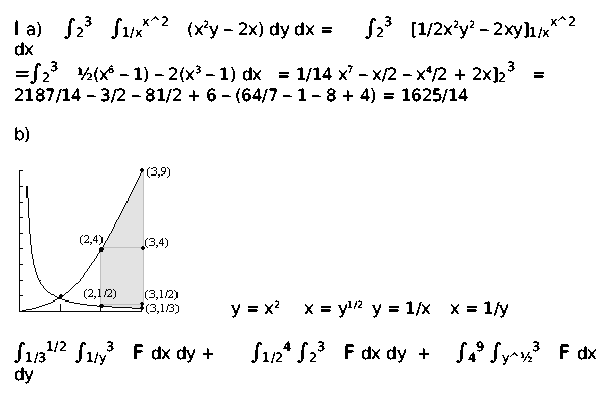



Answers To The Review Problems For The First Exam 251 05 10 In Spring 06




1 Consider The Curve Defined By X2 Xy Y2 27 A Write An




Implicit Differentiation



How To Use Implicit Differentiation To Find Dy Dx Unfortunately 2xy Y 2 1 Quora
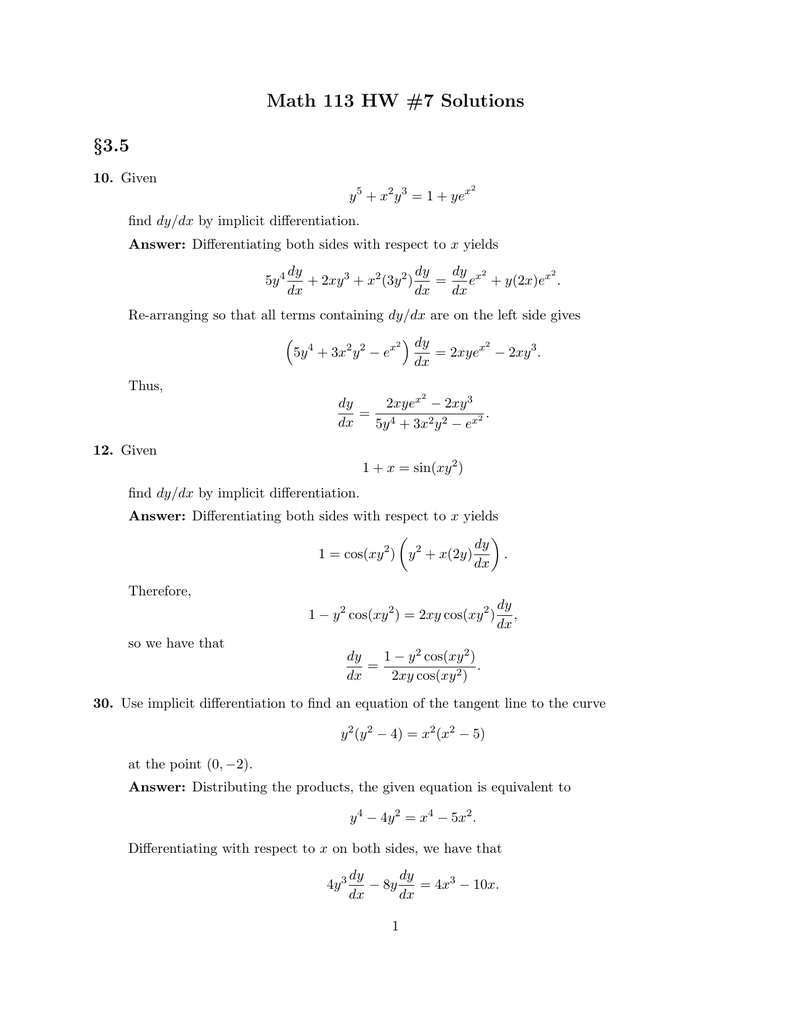



Math 113 Hw 7 Solutions 3 5




Find Dy Dx Of X 2 Xy Y 2 100 By First Principle Of Derivatives Brainly In




Engineering Mathematics Notes



4 2 Implicit Differentiation



Solved Find The Particular Solution Indicated For The Following Course Hero




Ppt Section 3 1 Powerpoint Presentation Free Download Id
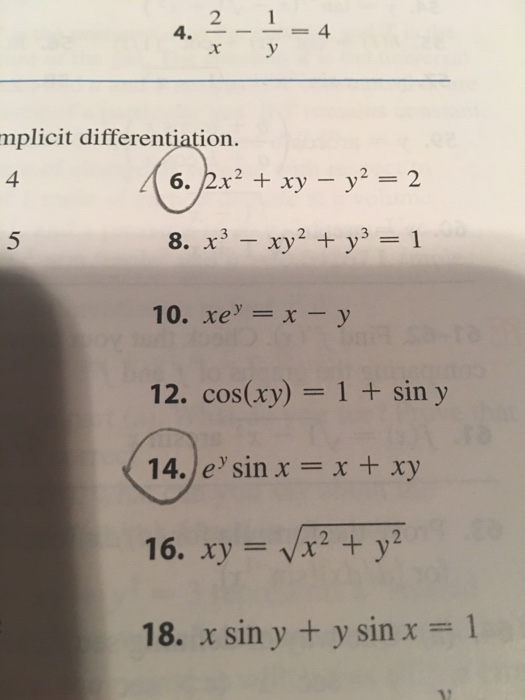



Solved Find Dy Dx By Implicit Differentiation 2x 2 Xy Chegg Com




If Y X Is The Solution Of Dy Dx X 2 1 Y 2 Y 0 2 Then Y 3 Homeworklib
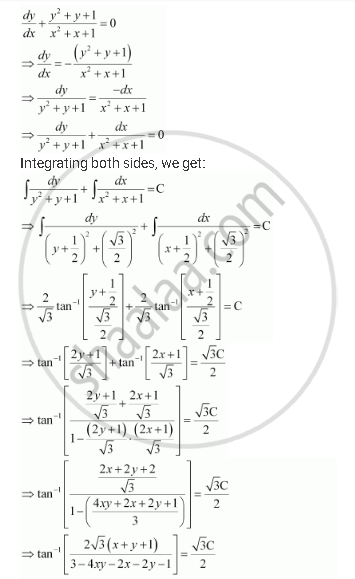



Show That The General Solution Of The Differential Equation Dy Dx Y 2 Y 1 X 2 X 1 0 Is Given By X Y 1 A 1 X Y 2xy Where A Is Parameter Mathematics Shaalaa Com



2



How To Find Dy Dx Of The Function Y X 1 X 2 X 1 2 Quora



How To Get The Special Solution Of This Differential Equation Y Xdy Dx 2 1 X 2 Dy Dx Where X 1 Y 1 Quora



Find Dy Dx Of X 2 Y 2 2 4xy Sarthaks Econnect Largest Online Education Community



Solve 2 Y 3 Xy Dy Dx 0 Given That Y 1 2 Studyrankersonline




Math 432 Hw 2 5 Solutions




Solve Y 2 2x 2y Dx 2x 3 Xy Dy 0 Mathematics Stack Exchange



Using Euler Rsquo S Method Find An Approximate Value Of Corresponding To 1 Given That Dy Dx X 2 Y And Y 1 When X 0 Use The Step Size H 0 25




Math 432 Hw 2 5 Solutions




If 2 X 2 Y 2 X Y Then Dy Dx Is Equal To 2 Y 2 X B 1 1 2 X 1 2 Y D 2 X 1 2 Y 2 Y 2 X 1




Find Dy Dx If X 2 2xy Y 2 0 Brainly In
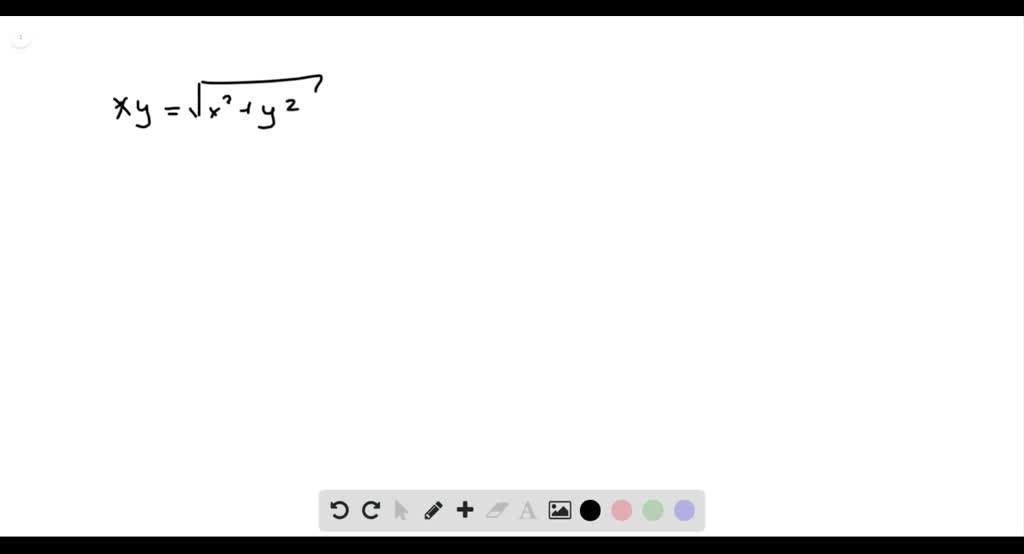



Solved Find Dy Dx By Implicit Differentiation Xy Sqrt X 2 Y 2




Implicit Differentiation


